MS in Physics, MA in Philosophy
PhD candidate in Computational Sciences
Doctoral researcher at TÜBİTAK Research Institute for Fundamental Sciences
PhD candidate in computational sciences at Kadir Has University
artun @ mail.com

/RESEARCH
Spin Glasses
Spin-Glass Phases in Complex Spin Systems
Spin-glass phases are phases in which the microscopic degrees of freedom are frozen in time but disordered in space, occurring in complex systems with frustrated interactions.
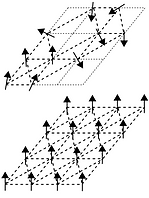
The left figure shows an illustration of the random spin structure of a spin glass (top) and the ordered one of a ferromagnet (bottom). The spin structure of the spin-glass is displaying different patterns of alignments in different regions. The spin configuration is dynamic and slowly changing due to significant relaxation times even more extensive than experimental observation timescales. In return, slowly relaxing magnetization can be observed. Furthermore, these systems may have very different equilibrium configurations, with different portions of the system being not alike. The degeneracy in free energy minima can be better seen with the notion of ground-state entropy, which can be injected into the system by bond randomness, thus frustration.
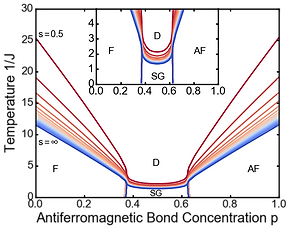
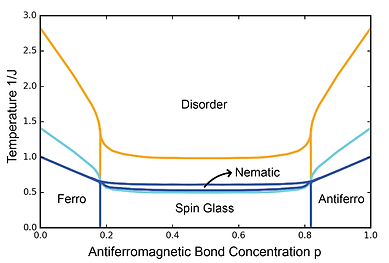
Thus far, the spin-glass phase has been studied on the simplest models, that is predominantly on the spin-1/2 Ising magnet. We have obtained, in spatial dimension d=3, the spin-glass phase and s-sequenced global phase diagram for all spins s>1/2 from an exact renormalization group calculation. For all spins s, we find and analyze chaotic behavior of the interactions under scale change.
The right figure shows the calculated phase diagrams of the spin-s Ising spin glasses in d=3.
Spin Glass Machine: The Complex Systems Classificator
We have developed a general classification (and therefore clustering) scheme for complex data. We have successfully tested this approach on multigeographic and multicultural music and on brain electroencephalograms in our first work, and we have been further applying our method to various complex data such as COVID-19 data, music, human brain activity via functional MRI, etc.
%20Spin-Glass%20Behavior%20in%20Frustrated%20Ising%20Models%20with.png)
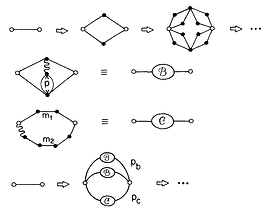
The idea behind the method is to use the chaos under scale change in spin glasses as a controllable and wide-ranged behavior for projection from complex systems. We used the fractality in complex data and in the spin-glass dynamics. Chaos under scale change is the essence of spin-glass ordering and can be obtained, continuously tailor-made, from the exact renormalization-group solution of Ising models (see the upper left figure) on frustrated hierarchical lattices (see the upper right figure). Chaotic renormalization-group trajectories of the standart chaotic hierarchical lattices exhibit fractal behavior. Various complex signals also exhibit fractal dynamics. Comparing the fractal spectra, we find the optimum fit in terms of spin-glass indices p, pb, pc, m1, m2. In the parameter space, spin-glass indices project the complex data.

Spin-Glass Sponges
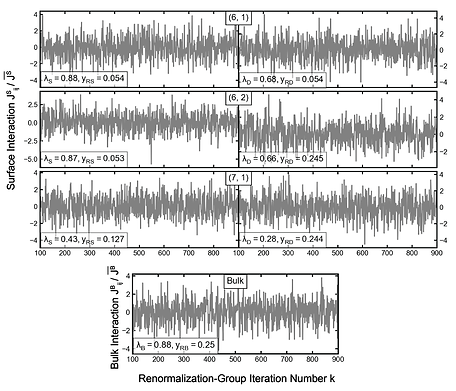
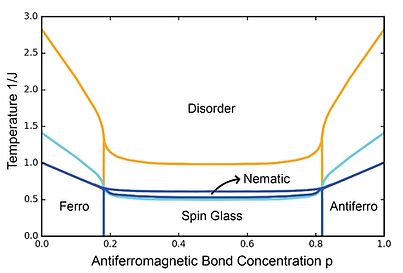
We identified a novel behavior in spin glasses, which we termed as 'spin-glass sponges'. We have studied surfaced spin-glass systems by exact renormalization-group theory of d=3 hierarchical lattices. We find that a flat surface does not support chaotic rescaling and therefore does not have a surface spin-glass phase. Therefore, we have introduced a new and physically realizable system, the spin-glass sponges, with higher fractal dimensional surfaces. We find that for higher spongevity, surface chaotic rescaling occurs even in absence bulk chaotic rescaling. We calculate phase diagrams with sole surface spin-glass phase, surface-and-bulk spin-glass phase, and disordered phase, all three phases meeting at a special multicritical point M. In fact, chaos in the sole surface spin-glass phase is stronger than in the bulk-driven surface spin glass.

Spin-Glass Phase under External Field
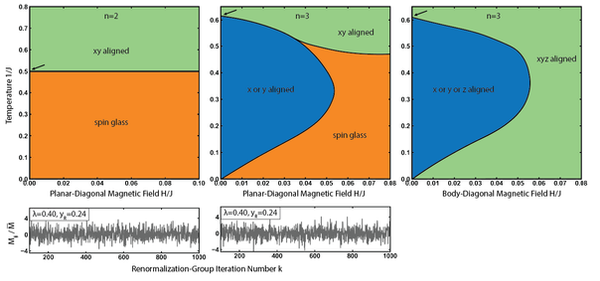
We discovered the presence of spin-glass phases under external magnetic fields. In our study on n-component cubic-spin systems, we observed a plethora of ordered phases under finite fields. Moreover, we demonstrated, for the first time, spin-glass phase under planar-diagonal and body-diagonal magnetic fields. This spin-glass phase results from the asymptotic competition of the +x or +y aligned phase and the xy alternating phase.
Liquid Crystal Phase in Spin Glasses
Liquid crystals are a distinct phase of matter that exhibit properties of both liquids and crystalline solids. In a liquid crystal, molecules have some degree of order similar to solid crystals, but they also possess the mobility and fluidity characteristic of liquids.
The nematic phase is one of the several phases observed in liquid crystals. In this phase, the molecules are ordered along a particular direction (referred to as the director), but they do not have a well-defined positional order. Instead, they exhibit long-range orientational order while maintaining fluid-like behavior in terms of their positional arrangement. The molecules in a nematic phase tend to align parallel to each other along the director axis but have no positional arrangement like the rigid structure found in a solid crystal.

We found a nematic phase in the n-component cubic-spin spin-glass system. This phase occurs between the low-temperature spin-glass phase and the high-temperature disordered phase for systems with a number of components n≥3. The results are derived from renormalization-group calculations, which are considered exact on a hierarchical lattice and approximate on the cubic spatial lattice. The nematic phase is found to completely intervene between the spin-glass phase and the disordered phase.
Criticality and Phase Transitions
Multicritical Orderings
Merging different systems may result in something new more than their sum as complexity suggests. We merged Potts and clock systems and calculated global phase diagrams in 2 and 3 spatial dimensions from renormalization-group solutions with the recently improved (spontaneous first-order detecting) Migdal-Kadanoff approximation or, equivalently, with hierarchical lattices with the inclusion of effective vacancies. Five different ordered phases are found which do not live in these systems separately.

Conventionally ordered ferromagnetic, quadrupolar, antiferromagnetic phases and algebraically ordered antiferromagnetic, antiquadrupolar phases. These five different ordered phases and the disordered phase are mutually bounded by first- and second-order phase transitions, themselves delimited by multicritical points: Inverted bicritical, zero-temperature bicritical, tricritical, second-order bifurcation, and zero-temperature highly degenerate multicritical points. One rich phase diagram topology exhibits all of these phenomena.
Nonequilibrium Phase Transitions
Dynamical systems are said to be out of equilibrium if the microscopic processes violate detailed balance. Roughly speaking, the term ‘nonequilibrium’ refers to situations where the probability currents between microstates do not vanish.
Equilibrium is more of an exception than the rule, and structural changes usually occur in nonequilibrium conditions. Thus there is much to be learned about the complex ordering phenomena occurring far from equilibrium. While we understood very little about the general aspects of nonequilibrium systems, the equilibrium critical phenomena have been much studied and have been shown to display universal features. This universality emerges from large-scale fluctuations in such a robust way that one can expect that similar mechanisms will work in nonequilibrium situations as well. Thus, investigating the similarities and differences of equilibrium and nonequilibrium orderings may help discover nonequilibrium systems' distinctive but still robust properties. An example to nonequilibrium phase transitions is the vanishing of metastable droplets. We studied existence and limits of metastable droplets applying renormalization-group theory.
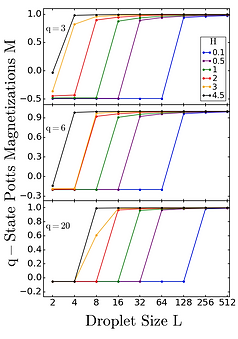
Equilibrium renormalization-group calculations can be extended to the calculation of the properties of metastable droplets of the non-equilibrium phase surviving inside the equilibrium thermodynamic phase.
We calculated the existence and limits of metastable droplets using finite-system renormalization-group theory, for q-state Potts models in spatial dimension d=3. The dependence of the droplet critical sizes on magnetic field, temperature, and number of Potts states q has been calculated. The same method has also been used for the calculation of hysteresis loops across first-order phase transitions in these systems. The hysteresis loop sizes and shapes have been deduced as a function of magnetic field, temperature, and number of Potts states q. The uneven appearance of asymmetry in the hysteresis loop branches has been noted. The method can be extended to criticality and phase transitions in metastable phases, such as in surface-adsorbed systems and water.
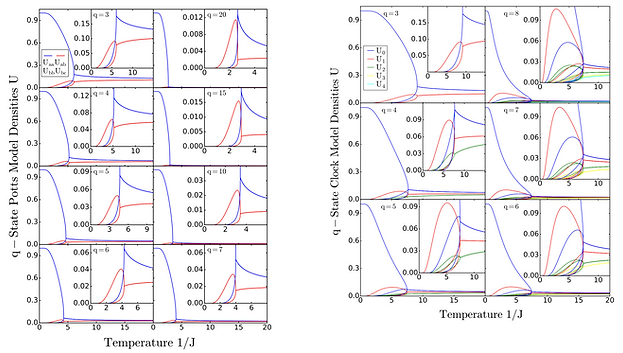
Total Thermodynamics at and away from Phase Transitions
Although originally introduced for critical phenomena, renormalization-group calculation gives the total thermodynamics of a system, at and away phase transitions. In order to effect this, the recursion relations of the local densities are needed, leading to calculation more complicated than that for phase boundaries and critical exponents.
We made this calculation for two families of systems, namely Potts and clock.
We calculated all local bond-state densities for q-state Potts and clock models in three spatial dimensions, d=3. We done the calculations by an exact renormalization group on a hierarchical lattice, including the density recursion relations, and simultaneously are the Migdal-Kadanoff approximation for the cubic lattice. We found reentrant behavior in the interface densities under symmetry breaking, in the sense that upon lowering temperature the value of the density first increases, then decreases to its zero value at zero temperature. For this behavior, we proposed a physical mechanism. We also found a contrast between the phase transition of the two models, and explained by alignment and entropy, as the number of states q goes to infinity. The above figure shows the calculated nearest-neighbor densities of the q-state Potts (on the left) and clock (on the right) models in d=3.
Complexity of Sociopolitical Systems
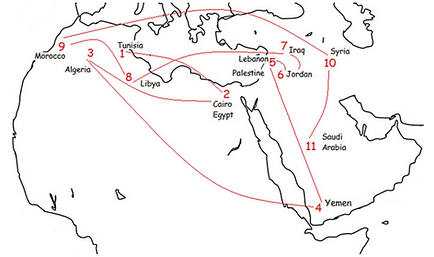
Self-Organized Criticality in Sociopolitical Movements
In today’s more connected, interdependent, fast, and highly globalized social world, conventional concepts and approaches for understanding social dynamics and social events have worsened day by day. There is a need for more dynamic points of view and new concepts that will help us grasp the underlying mechanisms of sociopolitical dynamics and what is happening beyond the phenomena that we observe as sociopolitical events. Complexity science offers a fresh understanding of natural systems since they are usually complex. In this study, we used the concept self-organized criticality gingerely to reinterpret the Arab Uprising. The self-organized criticality concept takes into account the whole society as a system. It interprets the so-called starting event not as an initial condition but rather as a tipping point where the system which has reached a critical state begins to reorganize itself into a new state—a phase transition takes place.
A system is said to be self-organizing, if the parts or actors of the system show an organized pattern at the macro-level. And, it is said to be critical when special conditions are effective. We present the said concept and reconsider it for its applicability to sociopolitical events. We argued over the Arab Uprising that how can (and should) we think of a sociopolitical system wherein big events can be triggered.
The right figure shows the spread of the protests in chronological order. The series of events were interconnected yet diverse, showing how the region is sensitive and how one part can be affected by another, whether it is a near neighbor or a far region—that shows the connectedness and interdependence over the region. Not only region but also it shows how the structure of each country is fragile in terms of economic, social, and political aspects. This nature of the region and countries may find its roots in the common culture, similar identities, common histories, shared and similar social memories, as well as also the connected and interdependent regional and global politics between the countries in the Middle East.
Complexity in Governance and Pandemic
The COVID-19 pandemic has inarguably been the most planet-shaking occurrence of modern memory. Furthermore, by imbibing to the differing social mores and differing governances of different countries, the pandemic has proceeded quite differently. This variegation has provided a distribution of archetypical complexity that requires communally vital understanding and analysis, which need be quantitative to be widely and quickly useful.
We applied newly developed complexity classification and clustering method, Spin Glass Machine, to COVID19-Governance correlations of 180 nations. Corruption, political stability, absence of violence/terrorism, voice and accountability, government response, and government-response time, as governance indicators, are correlated and clustered with COVID-19 cases and deaths between 1 January 2020 and 27 February 2022 in 180 countries. Systematic patterns and tell-tale clusterings are found to emerge.


Chaos and Complexity in Condensed Matter
Liquid Crystal in a Dirty Magnet
A nematic phase occurs in the n-component cubic-spin spin-glass system, between the low-temperature spin-glass phase and the high temperature disordered phase, for number of components n ≥ 3, in spatial dimension d = 3, thus constituting a liquid-crystal phase in a dirty (quenched-disordered) magnet. This result is obtained from renormalization-group calculations that are exact on the hierarchical lattice and, equivalently, approximate on the cubic spatial lattice. The nematic phase completely intervenes between the spin-glass phase and the disordered phase. The Lyapunov exponents, a measure of chaos, of the spin-glass chaos are calculated from n = 1 up to n = 12 and show odd-even oscillations with respect to n.

q-Statistics of Chaotic Behavior in Transient Current Through Thin Films
Statistics and dynamics are two separate and essential elements of physics near thermodynamic equilibrium. Under thermodynamic equilibrium conditions, Boltzmann-Gibbs (BG) statistical mechanics gives valid results as nature manifests itself as a Gaussian. Actually, under suitable approximations, using a master equation such as the Boltzmann transport equation, the laws of thermodynamics near-equilibrium can be derived. However, under conditions far from equilibrium, this does not work for most complex systems; statistics and dynamics must be unified. Constantino Tsallis' main attempt can be summarized in one sentence: He wanted to connect in theory our understanding in the sense of statistics of the macroscopic world and the microscopic world. Near equilibrium, where BG statistics works, we do not need to know the microscopic states of the subsystems of a system. We can calculate the entropy of the composed system macroscopically. However, for complex systems with shared characteristics such as long-range correlations, multifractality, and non-Gaussian distributions, and composability of the BG entropy disappears and becomes unuseful. Tsallis has enlarged the applicability domain of the frame of BG’s theory by extending the mathematical form of its entropy. Generalizing the mathematical form of the entropy has connected the microscopic world with its macroscopic appearance. Thus, far from equilibrium, the statistics of the dynamics follow the q-Gaussian generalization of the BG statistics or other more generalized statistics.
The q-distributions (see the right figure) of Tsallis q-statistics can be used for the characterization of chaos and multifractals. We used q-distributions to characterize the possible chaoticity within the transient current through thin films made of As2S2(Ag) and As2Se2(Al).

Chaotic Behavior in Transient Current Through Thin Films
Chaotic systems are dynamical systems whose apparently random states of disorder and irregularities are actually governed by underlying patterns and deterministic laws that are highly sensitive to initial conditions. Within the apparent randomness of chaotic complex systems, there are underlying patterns, interconnectedness, constant feedback loops, repetition, self-similarity, fractals, and self-organization.
Small differences in initial conditions, such as those due to measurement errors or rounding errors in numerical computation, can yield widely diverging outcomes for such dynamical systems, rendering long-term prediction of their behavior impossible in general. This can happen even though these systems are deterministic, meaning that their future behavior follows a unique evolution and is fully determined by their initial conditions, with no random elements involved. In other words, the deterministic nature of these systems does not make them predictable. This behavior is known as deterministic chaos or simply chaos.

The left figure shows that two trajectories varying 1 percent in initial conditions exhibit immensely different behaviors after some time.
It is essential to control chaos to build reliable devices, such as sensors, that use condensed matter materials to exhibit chaotic behavior under certain conditions.
We studied the possible chaotic behavior in the transient current through thin films made of As2S2(Ag) and As2Se2(Al). Such dielectric materials are known to show irregular current characteristics under constant electric field or voltage.
Quantum Electronics
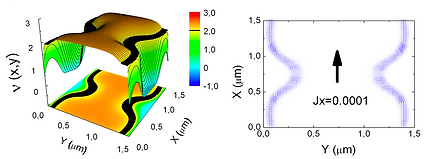
Transport Properties of Quantum Point Contacts (QPC)
Aside from studying the fundamentals of charge transport in mesoscopic conductors, QPCs can be used as sensitive charge detectors. Given quantum computation in solid-state systems, QPCs can be used as readout devices for the state of a qubit. A qubit or a quantum bit is a unit of quantum information in quantum computing. A QPC is simply a narrow constriction between two electrically conducting regions. QPCs are constructed on two-dimensional electron systems (2DES).
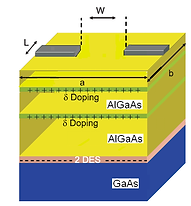
Via our current technology, we usually construct 2DES at the interface of a heterostructure (see the left figure). QPCs can be constructed either by inducing electrostatic potential on the plane of 2DES by depositing gates on the surface of the crystal and/or by chemically etching the structure. The small size of the constraint, that is, in a scale such that quantum mechanical effects can be observed, creates quantized energy levels in one dimension (perpendicular to the current direction). Therefore, the charge transport takes place depending on whether the electron's energy coincides with this quantized energy level. Ideally, at low bias voltages, if the energy of the electron is less than the lowest energy level of the constraint, no current can pass through the QPC. Otherwise, only a certain integer number of levels are involved, so the current is quantized.
Since the conductance through the QPC strongly depends on the size of the constriction, any potential fluctuation in the vicinity will affect the current through the contact. Single electrons can be detected with such a scheme. However, aside from the physical size of the QPC, the perpendicular component of the applied magnetic field, is another parameter that induces quantization on the 2DES. The exciting physics dictated by this quantization is observed as the quantum Hall effect. Due to the perpendicular magnetic field, the energy spectrum of the system is discrete, like that of a harmonic oscillator. These energy levels are known as the Landau levels (LLs). In the case of electrostatic equilibrium, when a fixed electron density is satisfied, the Fermi energy, Ef, can either be pinned to one of the LLs, where the system is compressible or can fall in between two successive LLs. In the second case, there is no equilibrium since there are no available states at the Ef for electrons to be redistributed. The equilibrium can be satisfied again; the system is known to be incompressible. Within these incompressible regions, the resistivity vanishes due to the absence of scattering. Hence all the applied current is confined to these regions.
We studied the transport in 2DES, and to control the current (in the future so that to use quantum information processing), we use the QPC and magnetic field. We tried to figure out the equilibration conditions.